Gautam Mohanty

B-School Forum, Odisha
Portfolio analysis is a mathematical algorithm created by the Nobel laureate Harry Markowitz during the 1950s. Markowitz portfolio analysis requires the following statistical inputs.
■ The expected rate of return, E(r), for each investment candidate (that is, every stock, every bond, etc.).
■ The standard deviation of returns, σ, for each investment candidate.
■ The correlation coefficients, ρ, between all pairs of investment candidates.
Markowitz portfolio analysis takes the statistical inputs listed above and analyzes them simultaneously to determine a series of plausible investment portfolios. The solutions explain which investment candidates are selected and rejected in creating a list of optimal portfolios that can achieve some expected rate of return. Each Markowitz portfolio analysis solution also gives exact portfolio weightings for the investment candidates in that solution.
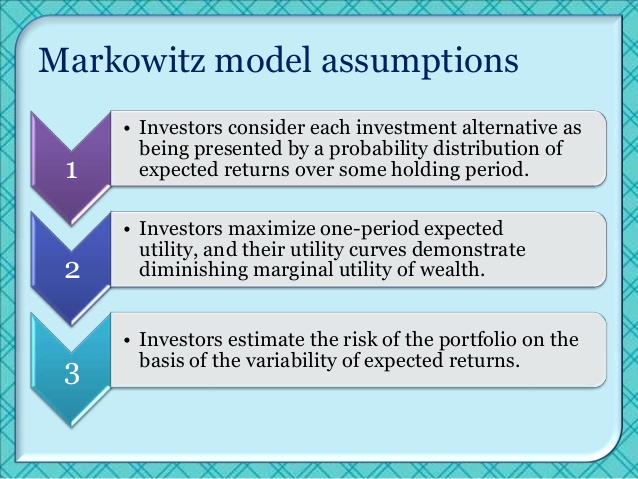
Basic Assumptions Portfolio theory is based on four behavioral assumptions.
- All investors visualize each investment opportunity (for instance, each stock or bond) as being represented by a probability distribution of returns that is measured over the same planning horizon (holding period).
2. Investors’ risk estimates are proportional to the variability of the returns (as measured by the standard deviation, or equivalently, the variance of returns).
3. Investors are willing to base their decisions on only the expected return and risk statistics. That is, investors’ utility of returns function, U(r), is solely a function of variability of return (σ) and expected return [E(r)]. Symbolically, U(r) =f[σ,E(r)]. Stated differently, whatever happiness an investor gets from an investment can be completely explained by σ and E(r).
4. For any given level of risk, investors prefer higher returns to lower returns. Symbolically, ∂U(r)/∂E(r) > 0. Conversely, for any given level of rate of return, investors prefer less risk over more risk. Symbolically, ∂U(r)/∂σ < 0. In other words, all investors are risk-averse rate of return maximizers.Reconsidering the Assumptions
The four behavioral assumptions just listed are logical and realistic and are maintained throughout portfolio theory. Considering the four assumptions implies the most desirable investments have:
■ The minimum expected risk at any given expected rate of return. Or, conversely,
■ The maximum expected rate of return at any given level of expected risk.
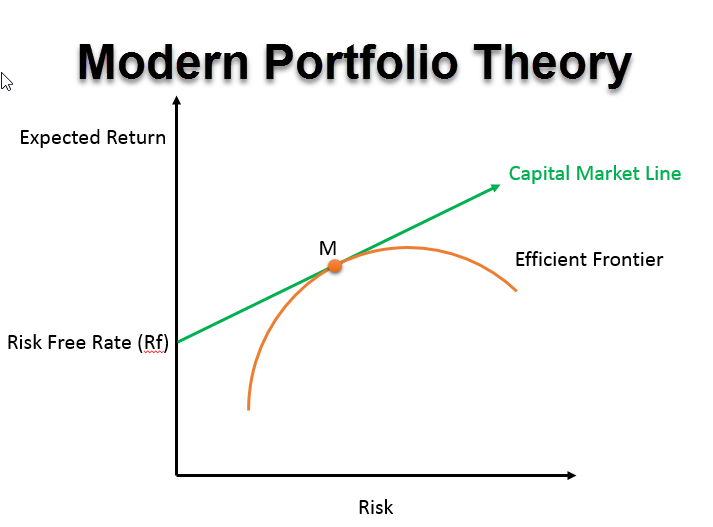
Investors described by the preceding assumptions will prefer Markowitz efficient assets. Such assets are almost always portfolios rather than individual assets. The Markowitz efficient assets are called efficient portfolios, whether they contain one or many assets.
If all investors behave as described by the four assumptions, portfolio analysis can logically (mathematically) delineate the set of efficient portfolios. The set of efficient portfolios is called the efficient frontier. The efficient frontier is the menu from which the investor should make his or her selection.
Before proceeding to the third step of the portfolio management process, portfolio selection, let us pause to reconsider the four assumptions listed previously. Portfolio theory is admittedly based on some simplifying assumptions that are not entirely realistic. This may raise questions in some people’s minds. Therefore, we will examine the validity of the four assumptions underlying portfolio theory.
The first assumption about probability distributions of either terminal wealth or rates of return may be violated in several respects. First, many investors simply do not forecast assets’ prices or the rate of return from an investment.
Second, investors are frequently heard discussing the ‘‘growth potential of a stock,’’ ‘‘a glamour stock,’’ or the ‘‘quality of management’’ while ignoring the investment’s terminal wealth or rates of return.
Third, investors often base their decisions on estimates of the most likely outcome rather than considering a probability distribution that includes both the best and worst outcomes. These seeming disparities with assumption 1 are not serious. If investors are interested in a security’s glamour or growth, it is probably because they (consciously or subconsciously) believe that these factors affect the asset’s rate of return and market value.
And even if investors cannot define rate of return, they may still try to maximize it merely by trying to maximize their net worth: Maximizing these two objectives can be shown to be mathematically equivalent. Furthermore, forecasting future probability distributions need not be highly explicit. ‘‘Most likely’’ estimates are prepared either explicitly or implicitly from a subjective probability distribution that includes both good and bad outcomes.
The risk definition given in assumption does not conform to the risk measures compiled by some popular financial services. The quality ratings published by Standard & Poor’s are standardized symbols like AAA, AA, A, BBB, BB, B, CCC, CC, and C. Studies suggest that these symbols address the probability of default. Firms’ probability of default is positively correlated with their variability of return. Therefore, assumption is valid.
As pointed out, investors sometimes discuss concepts such as the growth potential and/or glamour of a security. This may seem to indicate that the third assumption is an over simplification. However, if these factors affect the expected value and/or variability of a security’s rate of return, the third assumption is not violated either. The fourth assumption may also seem inadequate.
Psychologists and other behaviorists have pointed out to economists that business people infrequently maximize profits or minimize costs. The psychologists explain that people usually strive only to do a satisfactory or sufficient job. Rarely do they work to attain the maximum or minimum, whichever may be appropriate.
However, if some highly competitive business managers attain near optimization of their objective and other business managers compete with these leaders, then this assumption also turns out to be realistic.
All the assumptions underlying portfolio analysis have been shown to be simplistic, and in some cases overly simplistic. Although it would be nice if none of the assumptions underlying the analysis were ever violated, this is not necessary to establish the value of the theory.
If the analysis rationalizes complex behavior (such as diversification), or if the analysis yields worthwhile predictions (such as risk aversion), then it can be valuable in spite of its simplified assumptions. Furthermore, if the assumptions are only slight simplifications, as are the four mentioned previously, they are no cause for alarm. People need only behave as if they were described by the assumptions for a theory to be valid.





















